Marcus du Sautoy - What We Cannot Know

Before taking his life, he wrote what many regard as the first book that made inroads into predicting the behaviour of the dice as it rolls across the table. Although written around 1564, Liber de Ludo Aleae didn’t see the light of day until it was eventually published in 1663.

It was in fact the great Italian physicist Galileo Galilei who applied the same analysis that Cardano had described to decide whether to bet on a score of 9 or 10 when three dice are thrown. He reasoned that there are 6 × 6 × 6 = 216 different futures the dice could take. Of these, 25 gave you a 9 while 27 gave you a 10. Not a big difference, and one that would be difficult to pick up from empirical data, but large enough that betting on 10 should give you an edge in the long run.

The mathematical mastery of the dice shifted from Italy to France in the mid-seventeenth century when two big hitters, Blaise Pascal and Pierre de Fermat, started applying their minds to predicting the future of these tumbling cubes. Pascal had become interested in trying to understand the roll of the dice after meeting one of the great gamblers of the day, Chevalier de Méré. De Méré had challenged Pascal with a number of interesting scenarios. One was the problem Galileo had cracked. But the others included whether it was advisable to bet that at least one 6 will appear if a dice is thrown four times, and also the now famous problem of ‘points’.

Pascal entered into a lively correspondence with the great mathematician and lawyer Pierre de Fermat in which they tried to sort out the problems set by de Méré. With the throw of four dice, one could consider the 6 × 6 × 6 × 6 = 1296 different outcomes and count how many include a 6, but that becomes pretty cumbersome.

Instead, Pascal reasoned that there is a 5⁄ 6chance that you won’t see a 6 with one throw. Since each throw is independent, that means there is a 5⁄ 6× 5⁄ 6× 5⁄ 6× 5⁄ 6= 625⁄ 1296= 48.2% chance that you won’t get a 6 in four throws. Which means there is a 51.8% chance that you will see a 6. Just above an evens chance, so worth betting on.

The problem of ‘points’ was even more challenging. Suppose two players – let’s call them Fermat and Pascal – are rolling a dice. Fermat scores a point if the dice lands on 4 or higher; Pascal scores a point otherwise. Each, therefore, has a 50:50 chance of winning a point on any roll of the dice. They’ve wagered £64, which will go to the first to score 3 points. The game is interrupted, however, and can’t be continued, when Fermat is on 2 points and Pascal is on 1 point. How should they divide the £64?

Traditional attempts to solve the problem focused on what had happened in the past. Maybe, having won twice as many rounds as Pascal, Fermat should get twice the winnings. This makes no sense if, say, Fermat had won only one round before the game was interrupted. Pascal would get nothing but still has a chance of winning. Niccolò Fontana Tartaglia, a contemporary of Cardano, believed after much thought that it had no solution: ‘The resolution of the question is judicial rather than mathematical, so that in whatever way the division is made there will be cause for litigation.’

Others weren’t so defeated. Attention turned not to the past, but to what could happen in the future. In contrast to the other problems, they are not trying to predict the roll of the dice but instead need to imagine all the different future scenarios and to divide the spoils according to which version of the future favours which player.

It is easy to get fooled here. There seem to be three scenarios. Fermat wins the next round and pockets £64. Pascal wins the next round, resulting in a final round which either Pascal wins or Fermat wins. Fermat wins in two out of these three scenarios so perhaps he should get two-thirds of the winnings. It was the trap that de Méré fell into. Pascal argues that this isn’t correct. ‘The Chevalier de Méré is very talented but he is not a mathematician; this is, as you know, a great fault.’ A great fault, indeed!

Pascal, in contrast, was great on the mathematical front and argued that the spoils should be divided differently. There is a 50:50 chance that Fermat wins in one round, in which case he gets £64. But if Pascal wins the next round then they are equally likely to win the final round, so could divide the spoils £32 each. In either case Fermat is guaranteed £32. So the other £32 should be split equally, giving Fermat £48 in total.

Fermat, writing from his home near Toulouse, concurred with Pascal’s analysis: ‘You can now see that the truth is the same in Toulouse as in Paris.’

Pascal and Fermat’s analysis of the game of points could be applied to much more complex scenarios. Pascal discovered that the secret to deciding the division of the spoils is hidden inside something now known as Pascal’s triangle.

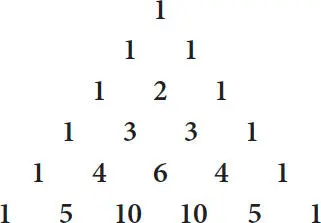
The triangle is constructed in such a way that each number is the sum of the two numbers immediately above it. The numbers you get are key to dividing the spoils in any interrupted game of points. For example, if Fermat needs 2 points for a win while Pascal needs 4, then you consult the 2 + 4 = 6th row of the triangle and add the first four numbers together and the last two. This is the proportion in which you should divide the spoils. In this case it’s a 1 + 5 + 10 + 10 = 26 to 1 + 5 = 6 division. So Fermat gets 26⁄ 32× 64 = £52 and Pascal gets 6⁄ 32× 64 = £12. In general, a game where Fermat needs n points to Pascal’s m points can be decided by consulting the ( n + m )th row of Pascal’s triangle.

There is evidence that the French were beaten by several millennia to the discovery that this triangle is connected to the outcome of games of chance. The Chinese were inveterate users of dice and other random methods like the I Ching to try to predict the future. The text of the I Ching dates back some 3000 years and contains precisely the same table that Pascal produced to analyse the outcomes of tossing coins to determine the random selection of a hexagram that would then be analysed for its meaning. But today the triangle is attributed to Pascal rather than the Chinese.

Pascal wasn’t interested only in dice. He famously applied his new mathematics of probability to one of the great unknowns: the existence of God.

‘God is, or He is not.’ But to which side shall we incline? Reason can decide nothing here. There is an infinite chaos which separated us. A game is being played at the extremity of this infinite distance where heads or tails will turn up … Which will you choose then? Let us see. Since you must choose, let us see which interests you least. You have two things to lose, the true and the good; and two things to stake, your reason and your will, your knowledge and your happiness; and your nature has two things to shun, error and misery. Your reason is no more shocked in choosing one rather than the other, since you must of necessity choose … But your happiness? Let us weigh the gain and the loss in wagering that God is … If you gain, you gain all; if you lose, you lose nothing. Wager, then, without hesitation that He is.

Called Pascal’s wager, he argued that the payout would be much greater if one opted for a belief in God. You lose little if you are wrong and win eternal life if correct. On the other hand, wager against the existence of God and losing results in eternal damnation, while winning gains you nothing beyond the knowledge that there is no God. The argument falls to pieces if the probability of God existing is actually 0, and even if it isn’t, perhaps the cost of belief might be too high when set against the probability of God’s existence.