Romeo Ortega - PID Passivity-Based Control of Nonlinear Systems with Applications

Consider the feedback system depicted in Figure 2.1, where is the nonlinear system (1), is the PID controller of 2.1 and is an external signal. Assume the interconnection is well defined.1 If the mapping is passive, the operator is ‐stable. More precisely, there exists such that

Figure 2.1Block diagram representation of the closed‐loop system of Proposition 2.1.

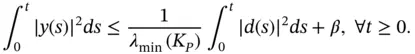
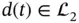From Proposition 2.1, we have that, for all signals , the output 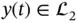. Under some additional assumptions, it also follows that 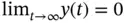. For instance, the latter property holds true, with 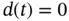, under the very weak assumption that there exists a steady‐state with and 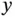constants. Invoking Theorem A.2 it follows that convergence of the output to zero is also achieved if 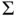is passive with a positive definite (with respect to the origin) storage function and the output is zero‐state detectable. Also, we note that the use of passivity in the design can potentially produce, in some cases, slow responses that cannot be improved due to limited tuning parameters, see, for instance, Section 4.5.

There are many important practical issues regarding PIDs that are not discussed in the book. For instance, the need to introduce an anti‐windup mechanism in the presence of saturation, limitations of the derivative gain, filtering the derivative action, and the use of other architectures of the PID. Also, the simplicity of the PID structure imposes limitations, for example on the set of unstable plants that can be stabilized with this class of controller and the difficulty of handling time delays and complex systems. For a detailed discussion of these, and other PID implementation issues, the interested reader is referred to Ang et al. (2005), Åstrom and Hägglund (1995, 2001), and Åstrom (2018).

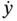As discussed earlier, it is necessary to ensure that the control law 2.1can be computed without differentiation nor singularities. The latter may arise due to the presence of the derivative term . Clearly, this term can be added only when the output 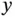has relative degree one, that is, when 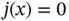. 2 But even for systems without a derivative action, a singularity may appear for systems with relative degree zero, that is with .

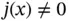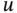The required well‐posedness assumptions in both cases are stated in the lemma below, whose proof follows immediately computing the expressions of for the closed‐loop system. As will become clear below, the assumptions are technical only, and rather weak, but they are given for the sake of completeness.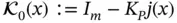

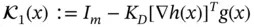
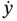If the system has relative degree zero, that is , the feedback system of Figure 2.1 with is well‐posed if the matrix

is full‐rank. On the other hand, if the system has relative degree one, that is and , the feedback system is well posed if the matrix

is full‐rank.

As a final comment of this section, we note that in van der Schaft (2016), PID control is viewed from a different perspective. Namely, assuming that is computable , it is shown that the closed‐loop system can be represented as a pH system with algebraic constraints . However, leaving aside the complexity of computing 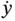, the stability analysis of this kind of systems remains an essentially open question.

In this section, we reveal a subtle aspect of the practical application of PID‐PBC, namely that for passive systems of relative degree one, there exists a steady state only if the energy extracted from the controller is zero at the equilibrium. The latter condition is known in PBC as dissipation obstacle and is present in many physical systems, for instance, all electrical circuits with leaky energy storing elements operating in nonzero equilibria – i.e. capacitors in parallel, or inductors in series, with resistors. Interestingly, this obstacle is absent in position regulation of mechanical systems since dissipation (due to Coulomb friction) is zero at standstill.

After briefly recalling the nature and mathematical definition of the dissipation obstacle, we prove the claim of inexistence of equilibria stated above in a more general context than just PID‐PBC, namely for all dynamic controllers incorporating an integral action on a passive output of relative degree one.

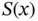To mathematically define the dissipation obstacle of a passive system with storage function , let us compute its derivative