George V. Chilingar - Acoustic and Vibrational Enhanced Oil Recovery

If the fracture surface is making random periodic vibrations in two mutually perpendicular directions in two mutually perpendicular directions lying on its surface, the liquid velocity in each of these directions are determined independent from one another. But, the aforementioned qualitative patterns remain valid. In particular, if periods of mutually perpendicular vibrations coincide, then the liquid particles’ trajectories at a sufficient distance from the fracture surface approach ellipses, and the size of these ellipses is exponentially declining. In a case when periods are substantially different, the liquid motion at distancing from the fracture surface approaches rectilinear harmonic vibrations coinciding in the direction and frequency with longer period vibrations. In a manner of speaking, the liquid layer has properties of a high frequency filter.

The quoted results are comparable with the solution of a problem of fading the agitations caused by a spherical fractured surface in the process of its harmonic vibrations along some direction in an incompressible liquid.

For instance, we will review for a case of Reynolds’ small numbers a spherical surface within which 95% of the total energy loss in a viscous incompressible liquid is dispersed ( Figure 2.4a). Assume that R 0is radius of vibration penetration and r 0is radius of the spherical surface. Then, the value δ = R 0− r 0may be considered the appropriate depth of vibrations’ penetration into the liquid. Then, correlation δ = R 0− r 0may be treated as the appropriate depth of vibrations’ penetration into the liquid. For the correlation δ / r 0vs. 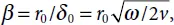the parameter we obtain a solid line diagram in Figure 2.4b. At that, 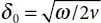is the same value with the dimension of length as in Equation (2.27). The same diagram within the considered limits of β variation may be approximated as follows:

(2.28)

(dashed line in Figure 2.3). Upon reducing by r 0, this equality is exactly equal to (2.27).

Thus, there is practically total coincidence of the results for a case of the flat and spherical fracture surfaces’ vibrations. The study result is also close if the agitation area from the spherical surface is defined not by the energy dissipation, but by leveling of the pressure field or by fading of the velocity field as it has been done for a case of a flat fracture. The agitation zone in the case of a spherical surface’s incremental advance in a viscous liquid under the problem’s conditions is by an order of magnitude greater in size than in the case of its vibration. This conclusion is in the qualitative agreement with correlations of Equations (2.27)and (2.28), which indicate an expansion of the agitation zone with the decline in the vibration frequency.

In conclusion, one may note that under the assumption of incompressibility of liquids, a case of lateral fracture surface vibrations is trivial as the liquid vibrates in this direction together with the fracture surface. If compressibility is present, then the process is described by a wave equation which includes an addend accounting for the dissipation of the vibration energy.

Figure 2.4 Vibration in a liquid volume at progressive vibrations of the fracture’s spherical surface.

Figure 2.5 Schematic advance of a mixture of a wetting and nonwetting liquids in a unit pore volume in a field of elastic vibrations. A rand A aare the relative and absolute displacement amplitudes of the nonwetting liquid; ε rand ε aare the relative and absolutes dislocations.

In the case of pore volume filled up with a wetting liquid with floating droplets of nonwetting liquid, the vibration penetration depth may be estimated treating the mixture equivalent to some liquid with viscosity greater than that of the wetting liquid with droplets of a nonwetting liquid. In order to estimate the absolute and relative amplitudes of displacements, force, and energy expense needed for providing vibrations in a reservoir, we will review a unit pore volume restricted by the rock matrix. Let us assume that a selected volume is filled up with a wetting liquid with floating droplets of a non-moisturizing liquid. To this volume are applied harmonic vibrations with a frequency ω with the maximum displacement amplitude A ( Figure 2.5). Walls of the pore volume and the wetting liquid are vibrating in phase, coherently with the displacement amplitude:

where t is the vibration time.

The simplest case is with nonwetting liquid droplets being balls of equal diameter d = 2 r suspended in the wetting liquid and the volume concentration C within the study volume of no greater than 5%. It such a case, the distances between the droplets are greater than 2 or 3 their diameters and their mutual influence may be disregarded. On assuming further that the Reynolds’ acoustic numbers are much smaller than a unit:

here, ν is the kinematic viscosity of the wetting liquid.

A solution for the nonwetting liquid displacement amplitude relative to the surrounding wetting liquid is [6]:

and the absolute amplitude of the displacement is

The force F needed for the occurrence of harmonic vibrations of such unit volume is:

where

ρ = ρ s− ρ ′ is the effective density of liquids’ mixture at vibration;

ρ s= ρ e(1 − C ) + Cρ n= ρ c[1 + C (Δ − 1)] is the static mixture density;

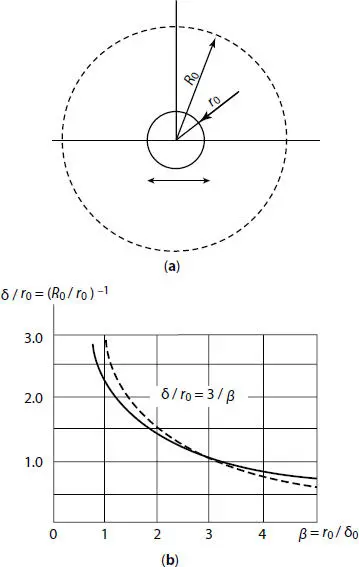
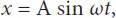
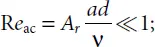
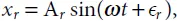
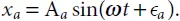
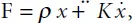
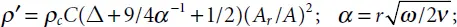
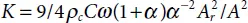is the effective vibration damping factor;