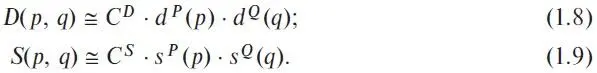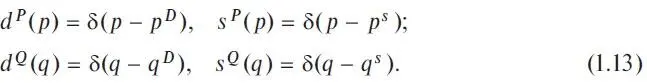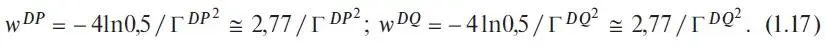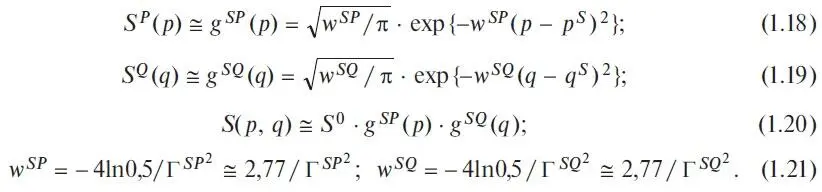Anatoly Kondratenko - Probabilistic Theory of Stock Exchanges

Let us briefly elaborate again on the rationale for this approach to physical-economic modeling. Market agents, forced to constantly work on the market in a continuously changing situation, are aware that the prices and quantities declared by them in the point strategy may not suit the counterparty at a given time. Based on previous experience in the market, they are well aware that they cannot know exactly how prices and quantities will develop in the market even in the near future. They are already used to operating in a market with great uncertainty, entailing high risks and the resulting potential costs. As a result, they realize that in the market they should always consider all their decisions and actions as possible with a certain degree of probability. This probabilistic aspect of the process of market decision-making is of great importance for understanding the behavior of agents in the market and the market as a whole [Mises, 2005; Gilboa et al., 2008]. Market agents think and act as homo oscillans . That is why they are forced to enter the real market not with discrete strategies, but with continuous strategies which can be represented by continuous probability distributions with certain widths correlated with the amount of uncertainty in the market situation in a given period of time.

As already noted, the description of continuous strategies requires the use of two-dimensional functions S&D, D ( p, q ), and S ( p, q ). Of course, it is rather tedious to calculate and analyze two-dimensional functions representing three-dimensional surfaces already in the case of a one-commodity market. For this reason, we will take one more step in simplifying our models, which will make it possible to perform economic calculations of real multi-agent markets and to analyze the results obtained by our method at the highest scientific level. Thus, we a priori assume that we can factorize the agent functions S&D with a sufficient degree of accuracy, i.e. we can approximate their representation as a product of one-dimensional functions as follows:

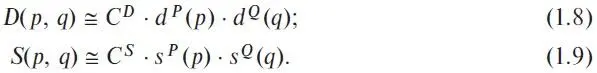
This type of factorization and the corresponding approximation, in which the price and quantitative variables are separated, will be called PQ -factorization and PQ -approximation, respectively. Here d P(p) and s P (p) are one-dimensional price functions, d Q(q) and s Q(q) are one-dimensional quantity functions of S&D normalized by definition to 1:

C D and C S are simply normalization factors. They are derived from the condition of such a natural normalization selection of the agent functions S&D:

Here D 0and S 0are obviously total demand and complete supply of the buyer and seller, respectively. Below we will also omit the word «total» for the sake of brevity. It is easy to show that the agent S&D-functions, D(p, q) and S(p, q) , normalized in this way, are dimensionless functions. It is also obvious that in the point or discrete strategy described above, one-dimensional functions are represented by the so-called Dirac delta functions as follows:

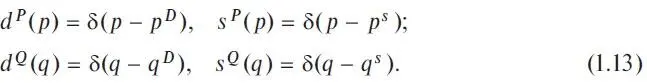
Keep in mind, that the special Dirac function by definition is zero everywhere except at the zero point, where it is equal to infinity, and its integral from minus infinity to plus infinity is 1. By the way, these functions can be applied to describe the probability functions of monopolist and monopsonist supply and demand in real markets.

Accounting for uncertainty in agents' strategies should obviously lead to «blurring» of these functions and turning them into continuous dome-shaped functions with maxima at the points p D, p S, q D и q S and agent widths Г DP, Г SP, Г SQ and Г DQ respectively. It seems reasonable, both from the economic and technical point of view, in the first approximation to use normal, or, simply, Gaussians distributions [Kondratenko, 2015]. Then the demand function has the following form in this approximation:

where the parameters w DPand w DQ (agent frequency parameters below) are related to the agent widths as follows:

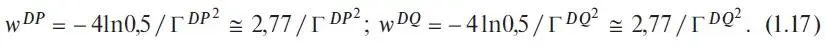
Formulas for demand have a similar structure naturally:

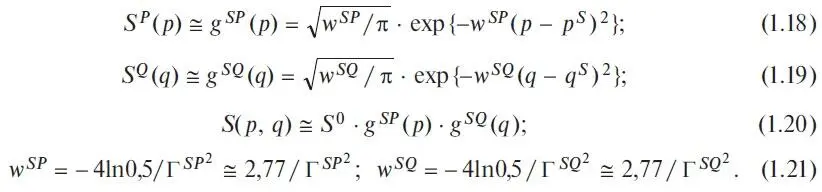
To avoid misunderstandings, note that formulas (1.17) and (1.21) express the relationship known for Gaussians between their agent frequency parameters and the widths, more precisely, the widths of Gaussians at half-height. The numerical values of these widths are set or selected explicitly or implicitly by the agents themselves, just as prices and quantities are set by them. But, very importantly, unlike price and quantity quotations, the «quotations» of widths are not explicitly exhibited either in negotiations or in organized markets. The values of these widths may not even be accurately realized by the agents themselves; in this respect, agents may act purely intuitively, depending on the market situation.

Summing up the intermediate results, we can briefly say that in this version of the theory the buyer's probabilistic demand function is described by four parameters, the price p D , the quantity q D and two widths Г DР and Г DР . The same statement is of course true for the seller's probabilistic supply function. It is these eight parameters that take into account all of the relevant market information that the buyer and seller use before they put up quotations at any given time in the process of trading in the market. And let us emphasize for clarity that usually both buyers and sellers declare or announce publicly and unambiguously only their price and quantity quotations, leaving the information about their widths "behind the scenes".

For our model grain market the probability functions S&D are presented graphically in Figs. 1.6–1.9.

Obviously, for a two-agent economy, all S&D market function surfaces have a simple smooth structure with one maximum. Of course, for more complex economies the structure of the surfaces will be much more complex.

Fig. 1.6. Graphical representation in the rectangular two-dimensional coordinate system [P, S& D] of one-dimensional price functions d P ( p ) and s P ( p ) as two-dimensional curves with maxima at prices p D and p S and widths Г DР and Г SР respectively. The values used for the widths are: Г DР = 23.8 $/ton, Г SР = 37.0 $/ton.