Mark Changizi - Harnessed - How Language and Music Mimicked Nature and Transformed Ape to Man

These eight-step-hill encounters are, I claim, a fundamental intermediate-level structure found in the pattern of Doppler pitches from human movers in our midst. Real movers will, of course, often diverge from this, but such instances should be viewed as deviations from this generic baseline. I call the generic encounter an intermediate-level structure because it is hierarchically above the individual “atoms” of movement (A, B, C, and D), and because full stories of movers in our midst may involve hundreds or thousands of footsteps—full stories of human movement are built by combining many short bouts of movement. Because the encounter is the generic short bout of movement, the generic long story of human movement is many eight-step hills—many encounters.

And now we are in a position to figure out whether these generic stories of human movement are found in music. In particular, we want to know if melodies are built out of encounter-like structures. And because generic encounters sound like eight-step pitch hills, we wish to see if melodies have any tendency to be built out of eight-beat pitch hills. Of course, we expect that any such tendency should be weak: the eight-step pitch hill is the expectation for the generic encounter, but you can be sure that composers like to tell nongeneric stories as well. Nevertheless, we hope to see the signs of the generic melody by looking across a great many melodies.

Eric Jordan and I set out to measure the average pitch contour for themes, following the lead of Professor David Huron, who first carried out measurements of this kind and found arches in average pitch contours. Themes were put into groups with other themes having the same number of notes; each theme’s pitches were normalized (so that the bottom and top of the tessitura were 0 and 1, respectively); and the average normalized pitch was computed across the group. Classical themes tend to have fewer than 25 notes, and in order to sample longer melodies, allowing us to better discern signs of eight-beat hills, we also measured from a set of 10,000 Finnish folk songs, which have themes with longer lengths of 25 to 40 notes. Figure 33 shows the average pitch contours for each group. There are, for example, 83 themes having exactly eight notes (the average of their contours is shown at the upper left in Figure 33). If melodies are built from eight-beat hills, as predicted, then we expect to see such hills in these averaged melodies. A casual glance across the average pitch contours for melodies having the same number of notes indicates a multiple-hill pattern in longer melodies. For themes with eight to 13 notes, only one hill is apparent in the average contour, but by 14 through about 19 notes, there is an apparent bimodality to the plots. Among the plots with 30 or more notes, a multiple-hill contour is strongly apparent. And the hills are very roughly eight notes in length. (These eight-note hills are due to themes with a predominance of quarter notes. Themes with a preponderance of eight notes lead to 16-note hills.)

Average Melodic Pitch Contour

Figure 33. Average melodic contour for melodies with the same number of notes. Thirty-two different plots are shown, for melodies having eight notes through 40 notes, shown by the number label along each x-axis. The “n” values show the number of melodies over which the average is calculated. If melodies are built with eight-beat hills, then because a sizable fraction of melodies consist mostly of beat-long notes, there should be a strong eight-note-hill tendency in these plots. There will also be sizable fraction of melodies consisting mostly of half-beat-long notes, and these will tend to have a sixteen-note hill. The smallest hills we expect to see, then, are eight-note ones.

To get a more quantitative estimate of the typical number of notes per hill in these average-melodic-contour data, Figure 34 plots the approximate number of hills as a function of the number of notes in the average melody. One can see that the number of hills is approximately 1/8 the number of notes, and so there are about eight notes per hill.

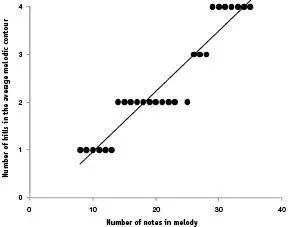
Figure 34. Number of hills in the average melodic contour as a function of the number of notes in the melody. The approximate number of arches from each plot in Figure 33 having eight notes through 36 notes (after which the number of hills is less clear) was recorded. The number of hills rises as 0.1256 times the number of notes in the melody. The number of notes per hill is thus 1/0.1256, which is 7.90, or approximately 8, consistent with our expectation from generic human encounters.

The data we have just discussed provide evidence of the “eightness” of melody’s hills. But recall that in order to show that they are hills , and not some other protuberance shape, we must show that the note durations at the start, peak, and end of the protuberance tend to have longer duration, just as hills are flatter on their ends and on top (see Figure 32 again). Focusing now just on classical themes with eight, nine, and ten notes, Eric Jordan and I determined the average duration (in beats) of each note. To generate a hill-shaped pitch contour, we would expect a “W”-shaped plot of how note durations vary over the course of a melodic theme, with longer durations at the start, middle, and end. Indeed, that’s what we found, as shown in Figure 35. Melodies tend to have longer-duration notes at the start and end (when the fictional mover is headed away from the listener), and also in the middle (when the mover is headed directly toward the listener).

Melodies appear to have a tendency to be built out of eight-beat hills, which is just what is expected if melody’s stories are about a fictional mover’s multiple encounters with the listener. Like arrival, interaction, and departure in eight steps, melodies tend to rise and fall over eight beats, and in the nonlinear fashion consistent with a real encounter. Melodies, in other words, seem to have the signature structure of stories built from Hello–HowAreYou–Good-byes.

In this and the previous sections we have seen that melody behaves in some respects like the Doppler pitch modulations of a person moving. But there’s much more to the similarity between Doppler and melody, and I discuss additional similarities in detail in the Encore. Here I will only hint at them, but I encourage you to read the hints, because they are exciting, and they are crucial to the case I am making.

Encore 3: “Fancy Footwork”This section will discuss how when people turn and their Doppler pitch changes, their gait can often become more complex. And I will provide evidence that music behaves in the same way. I referred to Encore 3 earlier in this chapter when we wrapped up the discussion of rhythm, because “Fancy Footwork” concerns how rhythm and pitch interact.

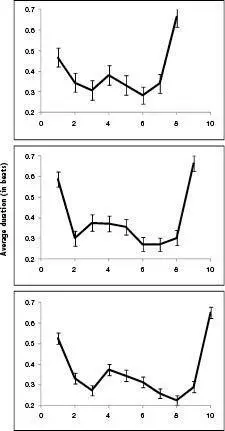
Figure 35. Average duration in beats of each note for classical themes with number of notes near that of the generic encounter (i.e., for 8, 9, and 10 notes). One can see the expected “W” shape, showing that the eight-note-hills in Figure 33 are truly hills .

Encore 5: “Home Pitch”This Encore section will discuss three similarities between melody and Doppler pitch modulations, each concerning how pitch distributes itself. Like Doppler pitch modulations, melodic contours have a fixed home range (called the tessitura); tend to distribute themselves uniformly over their home range; and tend to dwell longer at the edges of the pitch range.