Mark Haddon - The Curious Incident of the Dog in the Night-Time

And the dog sat in my lap and I stroked it.

And no one said anything for a while.

Then Father said, “Christopher, I would never, ever do anything to hurt you.”

Then no one said anything.

Then Mother came into the room and said, “You won’t be able to take him away with you, I’m afraid. The bed-sit’s too small. But your father’s going to look after him here. And you can come and take him out for walks whenever you want.”

And I said, “Does he have a name?”

And Father said, “No. You can decide what to call him.”

And the dog chewed my finger.

And then it was 5 minutes and the tomato alarm went. So Mother and I drove back to her room.

And the next week there was a lightning storm and the lightning hit a big tree in the park near Father’s house and knocked it down and men came and cut the branches up with chain saws and carried the logs away on a lorry, and all that was left was a big black pointed stump made of carbonized wood.

And I got the results of my maths A level and I got an A grade, which is the best result, and it made me feel like this:

And I called the dog Sandy. And Father bought him a collar and a lead and I was allowed to take him for walks to the shop and back. And I played with him with a rubber bone.

And Mother got flu and I had to spend three days with Father and stay in his house. But it was OK because Sandy slept on my bed so he would bark if anyone came into the room during the night. And Father made a vegetable patch in the garden and I helped him. And we planted carrots and peas and spinach and I’m going to pick them and eat them when they’re ready.

And I went to a bookshop with Mother and I bought a book called Further Maths for A Level and Father told Mrs. Gascoyne that I was going to take A-level further maths next year and she said “OK.”

And I am going to pass it and get an A grade. And in two years’ time I am going to take A-level physics and get an A grade.

And then, when I’ve done that, I am going to go to university in another town. And it doesn’t have to be in London because I don’t like London and there are universities in lots of places and not all of them are in big cities. And I can live in a flat with a garden and a proper toilet. And I can take Sandy and my books and my computer.

And then I will get a First Class Honors degree and I will become a scientist.

And I know I can do this because I went to London on my own, and because I solved the mystery of Who Killed Wellington?and I found my mother and I was brave and I wrote a book and that means I can do anything.

Question

Prove the following result:

A triangle with sides that can be written in the form n^2 + 1, n^2 – 1 and 2n (where n > 1) is right-angled.

Show, by means of a counterexample, that the converse is false.

Answer

First we must determine which is the longest side of a triangle with sides that can be written in the form n^2 + 1, n^2 – 1 and 2n (where n > 1)

n^2 + 1 – 2n = (n – 1)^2

and if n > 1 then (n – 1)^2 > 0

therefore n^2 + 1 – 2n > 0

therefore n^2 + 1 > 2n

Similarly (n^2 + 1) – (n^2 – 1) = 2

therefore n^2 + 1 > n^2 – 1

This means that n^2 + 1 is the longest side of a triangle with sides that can be written in the form n^2 + 1, n^2 – 1 and 2n (where n > 1).

This can also be shown by means of the following graph (but this doesn’t prove anything):

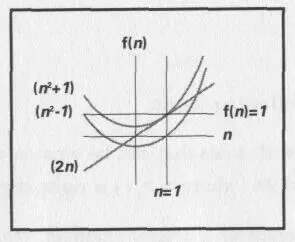
According to Pythagoras’s theorem, if the sum of the squares of the two shorter sides equals the square of the hypotenuse, then the triangle is right-angled. Therefore to prove that the triangle is right-angled we need to show that this is the case.

The sum of the squares of the shorter two sides is (n^2 – 1)^2 + (2n)^2

(n^2 – 1)^2 + (2n)^2 = n^4 – 2n^2 + 1 + 4n^2 = n^4 + 2n^2 + 1

The square of the hypotenuse is (n^2 + 1)^2

(n^2 + 1)^2 = n^4 + 2n^2 + 1

Therefore the sum of the squares of the shorter two sides is equal to the square of the hypotenuse and the triangle is right-angled.

And the converse of “A triangle with sides that can be written in the form n^2 + 1, n^2 – 1 and 2n (where n > 1) is right-angled” is “A triangle that is right-angled has sides whose lengths can be written in the form n^2 + 1, n^2 – 1 and 2n (where n > 1).”

And a counterexample means finding a triangle which is right-angled but whose sides cannot be written in the form n^2 + 1, n^2 – 1 and 2n (where n > 1).

So let the hypotenuse of the right-angled triangle ABCbe AB.

and let AB= 65

and let BC= 60

Then CA= v ( AB2– BC2) = v (652 – 602) = v (4225 – 3600) = v 625 = 25

Let AB= n^2 + 1 = 65

then n = v (65 – 1) = v 64 = 8

therefore (n^2 – 1) = 64 – 1 = 63 ? BC= 60 ? CA= 25

and 2n = 16 ? BC= 60 ? CA= 25

Therefore the triangle ABCis right-angled but it does not have sides which can be written in the form n^2 + 1, n^2 – 1 and 2n(where n > 1).

I found this in a book when Mother took me into the library in town in 1996.

This is not a metaphor, it is a simile, which means that it really did look like there were two very small mice hiding in his nostrils, and if you make a picture in your head of a man with two very small mice hiding in his nostrils, you will know what the police inspector looked like. And a simile is not a lie, unless it is a bad simile.

But I wouldn’t have Shreddies and tea because they are both brown.

Once I didn’t talk to anyone for 5 weeks.

When I was 6 Mother used to get me to drink strawberry-flavored slimming meals out of a measuring jug and we would have competitions to see how fast I could drink a quarter of a liter.

People say that you always have to tell the truth. But they do not mean this because you are not allowed to tell old people that they are old and you are not allowed to tell people if they smell funny or if a grown-up has made a fart. And you are not allowed to say “I don’t like you” unless that person has been horrible to you.

Stupid things are things like emptying a jar of peanut butter onto the table in the kitchen and making it level with a knife so it covers all the table right to the edges, or burning things on the gas stove to see what happened to them, like my shoes or silver foil or sugar.

I only did this once by borrowing the keys when she went into town on the bus, and I hadn’t driven a car before and I was 8 years old and 5 months so I drove it into the wall, and the car isn’t there anymore because Mother is dead.

It is permitted to move the chairs and the table in the kitchen because that is different, but it makes me feel dizzy and sick if someone has moved the sofa and the chairs around in the living room or the dining room. Mother used to do this when she did the hoovering, so I made a special plan of where all the furniture was meant to be and did measurements and I put everything back in its proper place afterward and then I felt better. But since Mother died Father hasn’t done any hoovering, so that is OK. And Mrs. Shears did the hoovering once but I did groaning and she shouted at Father and she never did it again.