Хелен Девитт - Some Trick - Thirteen Stories

Let’s take 2 people, A and B. A is a heroin addict. B’s idea of a narcotic is Earl Grey tea.

We take a randomly selected infant, toss a coin, and allocate it according to the result of the coin toss: Heads A, Tails B.

We repeat the procedure.

In 10 trials, the likeliest number of Heads is 5. If we run sequences of 10 trials, though, we shall sometimes have fewer than 5, sometimes more, and the distribution of Heads will follow the familiar Gaussian curve:

In 20 trials, the likeliest number of “successes” is 10 — that is, the Gaussian curve shifts to the right:

The two PDFs lodged awkwardly upside down at Peter’s edge of the table, jamming up against napkin dispenser, sugar pourer, salt & pepper shakers, red plastic bottle of ketchup, yellow plastic bottle of mustard.

When one gives a lecture or seminar, one does not have to do battle with condiments. He had not prepared for the contingency.

If we repeat the procedure on a daily basis, said Peter, ineffectually shifting the PDFs to give Jim a better view, the infant’s exposure to misallocation will tend to be rectified with relative frequency — though, on the other hand, the infant will never be guaranteed enjoyment of a good draw for very long. If the procedure is conducted weekly, more hangs on the result; if monthly, quarterly, yearly, more still.

He pressed the palms of his hands to his eyes.

Peter had written a book of robot tales with a happy beginning which had made, as it turned out, what seemed a lot of money, and yet not enough money to mitigate contractual relations with persons who had professed to love it yet sought to remove references to e iπ . He had explained that discovering e iπ at the age of 9 was the only thing that had kept him from suicide and been brushed indulgently aside.

The money had appeared to make it worth the while of Jim (said to be a “hot shot literary agent”) to represent Peter’s interests on a second book of robot tales. It had proved more complicated than had first appeared.

Jim did not know the secret of a happy beginning: My parents died when I was born.

Peter had made a special trip to New York to explain the binomial distribution in person to Jim. He wanted to reduce the likelihood of contractual obligation to persons like the persons he knew too well.

Now they sat in a booth in a diner.

Peter had suggested meeting in Jim’s flat, on the assumption that this would minimise the number of people in the vicinity mistakable for Jim.

Jim had held out for the diner.

Peter had suggested that Jim wear a yellow sweater for ease of identification.

Jim had not taken up the suggestion.

Jim was wearing a brown pullover and brown trousers. His hair and eyes were brown. For the whole of the walk from office to diner Peter had been terrified of losing sight of Jim in the crowd and then failing to recognise him again. The worry now was that if, for instance, Jim went to the men’s room and someone else came and sat down, Peter might fail to notice the difference. That was one worry, and another worry was that the name “Jim” might slip his mind, as names generally did.

The surface of the table was taken up with all sorts of paraphernalia superfluous, not to say impedimental, to ratiocination. Peter had done what he could to maximise the surface area available for display of illustrative materials; he had waived the offer of lunch. Jim had ordered something or other. He had exchanged badinage with the waitress.

We posit daily reassignment, Peter went doggedly on. As our number of trials approaches 100, the number of days of infant allocation to A comes to cluster, of course, around 50:

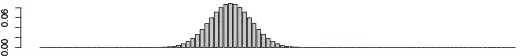
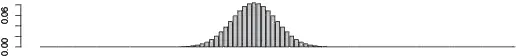
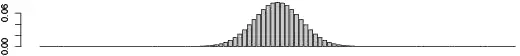
He had been up all night running variations in R on

par(mfrow=c(3,1))

barplot(dbinom(0:100,10,.5))

barplot(dbinom(0:100,20,.5))

barplot(dbinom(0:100,30,.5))

—all this before catching the 5 am AirBus to Gatwick from Gloucester Green, Oxford’s answer to Port Authority. In his haste he had, he realised, forgotten to label the x axis.

He would have liked, at this point, to throw in the towel, or rather retreat to his hotel and address the issue of the unlabelled x axis. But they were here. One must make the most of it.

Jim squirted ketchup placidly on crinkled fries. It seemed unlikely that a properly labelled x axis would have made the thing usefully clearer.

Perhaps Jim was one of the lucky ones. If he was an orphan he probably had all kinds of rosy notions.

But in fact , of course, persevered Peter — he had really been talking, understand, for only a minute or so, in a lecture he could have talked uninterrupted for an hour , with hand-outs , a blackboard — 50% of adults are not heroin addicts, so a model with a 50:50 chance of drawing one isn’t a very good fit. Suppose we try to get a feel for uneven odds.

You see, I suppose, that we can imagine a pool of 10 parents with 1 heroin addict and 9 tea-drinkers, or a pool with 9 heroin addicts and 1 tea-drinker. Instead of tossing a coin we draw, perhaps, a ball from an urn (containing, as it might be, 1 black ball 9 white or 9 black balls 1 white) and replace it before the next draw. In the first instance, over one hundred trials, the number of As — that is, draws of a heroin addict — would cluster around 10,