Benjamin Wardhaugh - Gunpowder and Geometry

This was self-publicity on a scale rare in Hutton’s century, or indeed in any. A good deal of money was involved – 600 subscriptions at fifteen shillings a book were not to be sneezed at – but the visibility had a value that could hardly be measured. It was rapidly becoming impossible to do mathematics, to like mathematics, to be aware of mathematics in Great Britain without knowing Charles Hutton’s name. Hutton was well advanced on the road from provincial schoolmaster with a taste for mathematical puzzles to national celebrity. He was aware of the change himself, of course. Throughout the 1760s he called himself ‘schoolmaster’ or ‘writing master’, but by the 1770s his title pages proclaimed him ‘author’; in 1772 he would switch to ‘mathematician’. And while Tonthu had been ‘of Newcastle’, Charles Hutton could call the town coldly ‘that part of the country in which I reside’, implying choice, impermanence, a lack of decisive ties to his provincial life.

What the subscribers to the Mensuration got for their money was a fat book which, despite a title that associated it with practical matters, was in fact a comprehensive treatment of theoretical as well as practical geometry – a substitute for Euclid’s Elements , indeed, as far as geometry was concerned. It began with definitions (‘A Line is a length conceived without breadth’), and it ran all the way up to the volumes of polyhedra and the areas contained under algebraically defined curves. Most of the book consisted of increasingly complex geometrical problems with rules for solving them: to find the area of a semicircle; to find the volume of a segment of a sphere; to find the surface area of a hyperboloid. Compared with the Guide , the emphasis was much less on carefully graded examples and much more on comprehensiveness, on a solid, gap-less treatment of a large body of material. It explained how to find the areas and the volumes of certain shapes and solids; how to construct certain curves and surfaces such as those arising from slicing a cone, or from rotating the conic sections that resulted (‘A conoid is a solid conceived to be generated from the revolution of the parabola or hyperbola about the transverse ax[is]’).

There was elegance and beauty here; there was also a tremendous display of learning. Hutton regularly succumbed to the temptation to exhibit his own cleverness at the expense of relevance or logical structure. Some of the more advanced, more difficult or more important solutions were backed up by proofs given in footnotes – and sometimes the footnotes were long, elaborate, even showy. Attached to a problem about right-angled triangles he indulged in not merely a demonstration but a ‘General Scholium’ (the term irresistibly recalls Isaac Newton’s use of it in his magnum opus, the Principia Mathematica ) with ‘some new theorems concerning the relations of the sides and angles of triangles’. These derived from infinite series – never-ending algebraic formulae – for the sine of an angle. A problem aimed at finding the circumference of a circle from its diameter and vice versa occasioned a note which set out the history of infinite series for the circular ratio from the seventeenth century onwards. Finding the length of part of an ellipse became the occasion for another, typical burst of complexity, with the full apparatus of geometry, algebra and calculus deployed to provide five different ways of solving the problem. Passages like this give an impression of extraordinary authorial dexterity: partly because they seem slightly out of place, obtruding upon the reader’s notice.

So this book, like the Guide , carefully fashioned Hutton himself. As seen in the pages of the Mensuration , he was a technically dextrous, extremely well-read mathematician, who could quote thinkers of the calibre of Newton as easily as obscure practical manuals of gauging and surveying. He was entitled to expect a lot of his readers: introducing calculus without explanation, stating rules without proofs because they were ‘too evident’ to need it, demanding faultless skill in imagining three-dimensional shapes and their manipulations.

But this was also someone who knew all about mathematical practice. The final section of the book turned to the practical applications of geometry, and worked through lengthy rules and examples for surveying, gauging liquid volumes, and measuring roofs, windows and chimneys. Hutton went to some trouble to emphasise his experience as a surveyor; in fact his first, last and only published survey – a map of Newcastle – appeared in the same year, 1770. He suggested improvements to practice, dismissed some instruments and praised others, and casually remarked on the best way of operating in certain situations. He urged the use of decimal, not duodecimal arithmetic by measurers, and he lambasted sellers of timber for their sharp practices, at rather petulant length (Hutton must have had a bad experience on this score; he wrote a letter to one of the Newcastle papers about it too).

This practical section culminated with the imagined exercise of planning and building a house. With columns at the front door and over six hundred feet of plaster mouldings, maybe the imagined house, a smart, ambitious Georgian edifice, bore some resemblance to the real one Hutton had planned and built for himself on Westgate Street.

Not everyone was convinced by Hutton’s posturing. There were some poor reviews in the London papers, by anonymous authors whose objections centred on his pretensions to practical knowledge. By and large, though, the reaction seems to have been positive. And the Mensuration stood the test of time; it would run to four editions, remaining in print until 1812. As late as 1830 a supporter would call it the work of a ‘masterly hand’, still ‘by far the best treatise on mensuration’ published in any country. One reader in Shropshire broke forth in verse to express his admiration:

O Science! trade and commerce are thy end,

By thee we import, and by thee we vend;

By thee we build our houses, till our lands,

And weigh and measure with unerring hands.

What art or rules could never yet display,

Nor all the rules of Science till this day

Were able to disclose [by] genius’ force,

Thy true-born son hath traced to the Source.

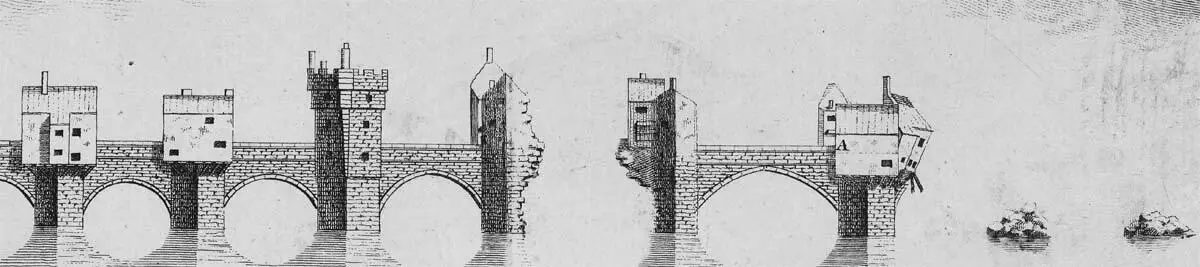
Newcastle bridge in ruins.

Hutton’s daily round of work must have been frenetic during these years in Newcastle around 1770. To teaching and administering a school, together with a continuing programme of reading and self-improvement, were now added the demands of writing and seeing through the press a steady flow of publications. Mathematics is hard to proof-read, and a geometry book like the Mensuration also required hundreds of diagrams to be commissioned and checked. A young Newcastle engraver named Thomas Bewick, subsequently a celebrity himself for – particularly – his engravings of birds, did some of his first work on the Mensuration , and he remembered that Hutton frequently came into his work room to inspect what he was doing. Despite Hutton’s increasingly assertive public persona, he found him ‘grave or shy’ in this private setting.

Hutton remained capable of a misstep. Late in 1771 the bridge at Newcastle was partly destroyed by a flood, massively disrupting travel in the city and the area. While an inquiry was taking place and before a replacement had been decided on, Hutton rushed into print – it’s hard to see how he got the text through the press as fast as he did – with a 102-page book on the theory of bridge building. It was an able, even a learned work, full of geometrical diagrams and cutting-edge mathematical analysis using some very clever calculus; the voice was recognisably the same self-confident mathematician of the Mensuration . The chief problem tackled was to find what shape the upper curve of a bridge should be for a given lower curve – or vice versa – in order to ensure its theoretical equilibrium. Hutton found some neat results and expressed a decided preference for a curve of his own invention, related to the catenary (the shape of a hanging rope or chain).