Allen Hunt - Hydrogeology, Chemical Weathering, and Soil Formation

Soil may be viewed as a one‐dimensional, two‐dimensional, or three‐dimensional object. The soil profile concept, introduced to western soil scientists by Curtis F. Marbut in 1921 (see Tandarich et al., 2002; Brevik et al., 2016), is one dimensional, defining soil as the vertical cross‐section from the surface downward through all the soil horizons and into the parent material. It was adopted as the basic unit for soil survey. During the 1920s and first half of the 1930s, soil surveyors mapped the spatial distribution of soils and in doing so were assuredly aware that soils were part of landscapes, but they did not consider soil as a three‐dimensional functional landscape unit. Concepts of that nature appeared first with the two‐dimensional soil catena and later with the three‐dimensional soil landscape, both of which, along with the soil profile, were to lend themselves to a systems approach.

As explained above, Simonson (1959) suggested the concept of the soil profile as an open system. Quantitative models of soil profile development followed. An example was the CENTURY model used to simulate the formation of soil organic matter (Parton et al., 1987). It established statistical relationships between production and decomposition rates of soil organic matter and four driving variables (annual precipitation, temperature controls on decomposition, soil texture, and plant lignin content) and then predicted soil carbon and nitrogen levels for 24 grassland locations in the Great Plains of the USA. Donald Sasscer and his colleagues (1971) built a deterministic model to study stable and titrated water through an old‐field system in Argonne, Illinois; the system consisted of a vegetation compartment and 49 soil compartments, each 1 cm thick. Jerry Kline (1973) used the same basic model to investigate soil–plant relationships and soil genesis.

Donald Lee Johnson (1985) devised an evolutionary model of profile pedogenesis that considered soil thickness changes:

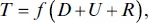
where T is soil thickness, D is profile deepening, U is soil upbuilding, and R is soil removal. An important feature of this model, and what makes it “evolutionary,” is that soil thickness is dynamic and may ebb or flow over time. This model formed the basis of Johnson and Watson‐Stegner’s (1987) comprehensive soil evolution model, which suggested that phenomena such as erosion, deposition, and pedoturbation can influence the soil formation processes. The model posited that soils may evolve along either progressive or regressive pathways:

where S is soil; P is progressive pedogenesis and includes processes, factors, and conditions that promote differentiated profiles; and R is retrogressive pedogenesis and includes processes, factors, and conditions that promote simplified profiles. This model was an attempt to allow for the fact that soil evolves in an ever‐changing environment so that polygenetic soils are the norm. Its keynote is polygenesis and stands in antithesis to monogenetic models and notions of zonal soils, normal soils, and climax soils.

Johnson and his colleagues (1990) developed a related evolutionary model. In summary, it assumed that

where s is a soil property or the degree of pedogenesis, D is a set of dynamic vectors and dD / dt their rate of change through time, P is a set of passive vectors and dP / dt their rate of change through time. The dynamic vectors include energy fluxes, mass fluxes, the frequency of wetting and drying events, organisms, and pedoturbation. The passive vectors include parent material, the chemical environment of the soil, permanently low water tables, the stability of slopes, and pedogenetic accessions such as fragipans, natric horizons, and histic horizons.

Jonathan D. Phillips (1993a) explored the idea of progressive–regressive pedogenic changes in his own numerical model that incorporated relative rates of progressive and retrogressive pedogenesis and feedbacks between the rate and the degree of soil development:

where sis soil development (degree of pedogenic alternation and profile organization), P is progressive pathways, and R is regressive pathways. The time differentials of P and R are defined as follows:

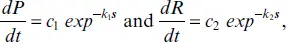
where c 1is the maximum rate of progressive pedogenesis and k 1a coefficient describing the decrease in P rates as soil develops; c 2is the maximum rate of regressive pedogenesis and k 2a coefficient describing the decrease in R rates as soil develops (negligible). Phillips’s analysis revealed that soil development may display deterministic chaos, with soil profile state at any time being unique to the interplay of progressive and retrogressive soil‐forming processes and sensitive to initial conditions, rather than simply the age of the deposit in which the soil is formed. This possibility challenges the classic view of soil developing unidirectionally to an increasingly differentiated state. Rather, it shows that pedogenesis can proceed in different directions due internal dynamics and thresholds, a point first made by Daniel Muhs (1982, 1984; see also Phillips, 1993b, 2001, 2013, 2017; Chadwick & Chorover, 2001).

In 1935, Geoffrey Milne, inspired by an idea of his colleague W. S. Martin (Brown, 2006), put forward the concept of the catena as a unified framework within which to study functional aspects of soils on hilly terrain. Milne was based at the East African Agricultural Research Station, Amani. A problem he faced was to map on a small piece of paper the complex entanglement of soils in a large piece of country. To surmount the problem, he took advantage of the fact that in many parts of East Africa, the topography over large tracts consists of little else but a repetition of crests and hollows, and a transect running from crest to hollow traverses very different soil profiles. Mapping the individual soils along the transect would be impossible in all but the most detailed of surveys. Nor, he reasoned, would it be necessary to do so because “they are not, properly speaking, individual soils at all, but are a compound soil unit of another kind in which a chain of profile‐differences occurs in a regular manner” (Milne 1935a, 192).

Milne used the term catena (the Latin word for “chain”) to describe the regular repetition of soil profiles on crest–hollow topography. He considered adopting the word suite , as used by Gilbert Wooding Robinson (1936) to describe a range of differing soils related by topography in Wales. Robinson confined his suites to soils formed in the same parent material; he did not deal with such extreme differences in soils on hills and in valleys as Milne did. In summary, Milne proposed the term catena to describe the lateral variation of soils on a hillslope and reasoned that, owing to the agency of geomorphological and pedological processes, all soils occurring along a hillslope are related to one another. He was quite explicit that the topographic relationships of the soils were the prime concern, and that the uniformity of parent material was of subsidiary interest.