John Crisp - Introduction to Microprocessors and Microcontrollers

MPU and CPU

An MPU is a MicroProcessor Unit or microprocessor. A CPU is a Central Processing Unit. This is the central ‘brain’ of a computer and can be (usually is) made from one or more microprocessors. The IBM design for the ‘Blue Gene’ supercomputer includes a million processors!

Remember:

MPU is the thing

CPU is the job.

Micro

The word micro is used in electronics and in science generally, to mean ‘one-millionth’ or 1×10 –6. It has also entered general language to mean something very small like a very small processor or microprocessor. It has also become an abbreviation for microprocessor, microcomputer, microprocessor-based system or a micro controller – indeed almost anything that has ‘micro’ in its name. In the scientific sense, the word micro is represented by the Greek letter μ (mu). It was only a small step for microprocessor to become abbreviated to μP.

Some confusion can arise unless we make sure that everyone concerned is referring to the same thing.

In each case, choose the best option.

1 A microprocessor:

(a) requires fuel, water and electricity.

(b) is abbreviated to μc.

(c) is often encapsulated in plastic.

(d) is never used in a CPU but can be used in an MPU.

2 A system must include:

(a) an input, an output and a process.

(b) something to do with a form of transport.

(c) a microprocessor.

(d) fuel, water and electricity.

3 All systems generate:

(a) movement.

(b) chips.

(c) waste heat.

(d) waste gases.

4 An MPU:

(a) is the same as a μP.

(b) can be made from more than one Central Processing Unit.

(c) is a small, single chip computer.

(d) is an abbreviation for Main Processing Unit.

5 Integrated circuits are not :

(a) called chips.

(b) used to construct a microprocessor-based system.

(c) solid state circuits.

(d) an essential part of an engine.

Unlike us, microprocessors have not grown up with the idea that 10 is a convenient number of digits to use. We have taken it so much for granted that we have even used the word digit to mean both a finger and a number.

Microprocessors and other digital circuits use only two digits – 0 and 1 – but why? Ideally, we would like our microprocessors to do everything at infinite speed and never make a mistake. Error free or high speed – which would you feel is the more important?

It’s your choice but I would go for error free every time, particularly when driving my car with its engine management computer or when coming in to land in a fly-by-wire aircraft. I think most people would agree.

So let’s start by having a look at one effect of persuading microprocessors to count in our way.

If the input of a microprocessor is held at a constant voltage, say 4 V, this would appear as in Figure 2.1.

Figure 2.1 A constant voltage

If we try to do this in practice, then careful measurements would show that the voltage is not of constant value but is continuously wandering above and below the mean level. These random fluctuations are called electrical noise and degrade the performance of every electronic circuit. We can take steps to reduce the effects but preventing it altogether is, so far, totally impossible. We can see the effect by disconnecting the antenna of our television. The noise causes random speckles on the screen which we call snow. The same effect causes an audible hiss from the loudspeaker. The effect of noise is shown in Figure 2.2.

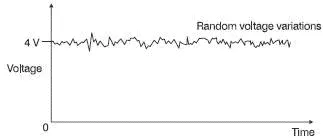
Figure 2.2 A ‘noisy’ voltage

Most microprocessors use a power supply of 5 V or 3.3 V. To keep the arithmetic easy, we will assume a 5 V system.

If we are going to persuade the microprocessor to count from 0 to 9, as we do, using voltages available on a 5 V supply would give 0.5 V per digit:

0 = 0 V

1 = 0.5 V

2 = 1 V

3 = 1.5 V

4 = 2 V

5 = 2.5 V

6 = 3 V

7 = 3.5 V

8 = 4 V

9 = 4.5 V

If we were to instruct our microprocessor to perform the task 4 + 4 = 8, by pressing the ‘4’ key we could generate a 2 V signal which is then remembered by the microprocessor. The + key would tell it to add and pressing the ‘4’ key again would then generate another 2 V signal.

So, inside the microprocessor we would see it add the 2 V and then another 2 V and, hence, get a total of 4 V. The microprocessor could then use the list shown to convert the total voltage to the required numerical result of 8. This simple addition is shown in Figure 2.3.

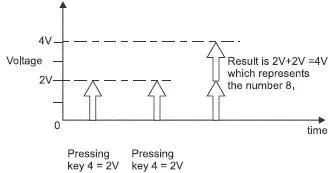
Figure 2.3 It works! 4 + 4 does equal 8

This seemed to work nicely – but we ignored the effect of noise. Figure 2.4 shows what could happen. The exact voltage memorized by the microprocessor would be a matter of chance. The first time we pressed key 4, the voltage just happened to be at 1.5 V but the second time we were luckier and the voltage was at the correct value of 2 V.

Figure 2.4 Noise can cause problems

Inside the microprocessor:

1.5 V + 2 V = 3.5 V

and using the table, the 3.5 V is then converted to the number 7. So our microprocessor reckons that 4 + 4 = 7.5!

Since the noise is random, it is possible, of course, to get a final result that is too low, too high or even correct.

Sorry, just dreaming. There isn’t one. The small particle-like components of electricity, called electrons, vibrate in a random fashion powered by the surrounding heat energy. In conductors, electrons are very mobile and carry a type of electrical charge that we have termed negative. The resulting negative charge is balanced out by an equal number of fixed particles called protons, which carry a positive charge (see Figure 2.5).

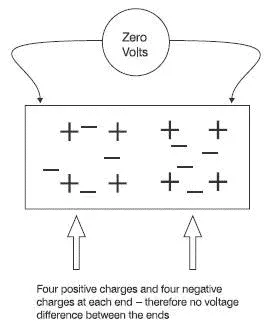
Figure 2.5 Equal charges result in no overall voltage

The overall effect of the electron mobility is similar to the random surges that occur in a large crowd of people jostling around waiting to enter the stadium for the Big Match. If, at a particular time, there happens to be more electrons or negative charges moving towards the left-hand end of a piece of material then that end would become more negative, as shown in Figure 2.6. A moment later, the opposite result may occur and the end would become more positive (Figure 2.7). These effects give rise to small random voltages in any conductor, as we have seen.

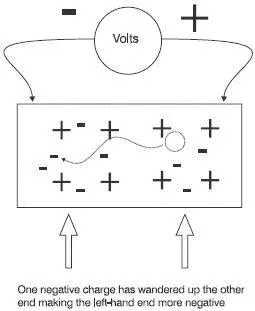
Figure 2.6 A random voltage has been generated

Figure 2.7 The opposite effect is equally likely